Difference between revisions of "Poisson's ratio"
| (One intermediate revision by the same user not shown) | |||
| Line 46: | Line 46: | ||
|ASTM D696 | |ASTM D696 | ||
|} | |} | ||
| + | |||
| + | |||
| + | '''Material Properties of Thermoplastic Pipe'''<br clear=all> | ||
| + | Creep modulus varies by plastic material, temperature, age, and time of loading ('''TI'''). The time of loading is defined as the period of time a plastic siphonic piping system is expected to be operating at the relevant pressure. Once the storm event is over and the system drains, the material recovers and assumes its original tensile modulus (i.e., '''EC(TI= 0) = Et'''). However, the material elastic modulus and creep modulus also typically decrease with age. Refer to pipe manufacturer data for the appropriate creep modulus value. | ||
| + | |||
Calculation of allowable pressure <math>(Pa)</math>: The minimum required pipe wall thickness <math>(t)</math> shall be based on '''Equations 8.1''' and '''8.2''' in '''Table 8.2''' and the mechanical and dimensional properties of the pipe material evaluated. '''Equations 8.1''' and '''8.2''' apply for a long cyclindrical tube of length '''L''' and wall thickness '''t''' under uniform external pressure with <math>(R/t) /> 10</math>. | Calculation of allowable pressure <math>(Pa)</math>: The minimum required pipe wall thickness <math>(t)</math> shall be based on '''Equations 8.1''' and '''8.2''' in '''Table 8.2''' and the mechanical and dimensional properties of the pipe material evaluated. '''Equations 8.1''' and '''8.2''' apply for a long cyclindrical tube of length '''L''' and wall thickness '''t''' under uniform external pressure with <math>(R/t) /> 10</math>. | ||
| Line 64: | Line 69: | ||
|} | |} | ||
<br clear=all> | <br clear=all> | ||
| − | The parameter <math>E_c</math> is the elastic creep modulus at the specified time of loading. The allowable pressure <math>(Pa)</math> shall be the calculated critical buckling pressure divided by the factor of safety (FS) as in equation below: | + | The parameter <math>E_c</math> is the elastic creep modulus at the specified time of loading. The allowable pressure <math>(Pa)</math> shall be the calculated critical buckling pressure divided by the factor of safety ('''FS''') as in equation below: |
'''Equation 8.3:''' | '''Equation 8.3:''' | ||
| Line 71: | Line 76: | ||
<math> P_a = \frac{P_cr}{FS} \ge 14.7 psia (1.0 bar)</math> | <math> P_a = \frac{P_cr}{FS} \ge 14.7 psia (1.0 bar)</math> | ||
<br clear=all> | <br clear=all> | ||
| − | Poisson's ratio is | + | |
| − | + | ||
| + | *'''Poisson's ratio''' is the ratio of the relative contraction strain (or transverse strain) normal to the applied load - to the relative extension strain (or axial strain) in the direction of the applied load <ref>http://www.engineeringtoolbox.com/poissons-ratio-d_1224.html</ref> | ||
Poisson's Ratio can be expressed as; | Poisson's Ratio can be expressed as; | ||
| Line 89: | Line 95: | ||
μ = Poisson’s Ratio (dimensionless) | μ = Poisson’s Ratio (dimensionless) | ||
| − | * | + | * '''Poisson's ratio''' describes the relative change of the lateral dimension of an object when the load is applied on the longitudinal direction and vice versa. (See reference video link [https://www.youtube.com/watch?v=hBnzrBhnzVo]) |
| − | |||
== References == | == References == | ||
Latest revision as of 15:05, 3 October 2017
Poisson ratio is one of the important criteria to be considered in pipe selection where the system may be subjected to sub-atmospheric pressure (e.g. Siphonic drainage systems). In general, a lower value in Poisson ratio provides better strength against collapse of pipe due to sub-atmospheric pressure.
In evaluating the limiting or maximum pressures, forces and deflections on plastic pipe, fittings and components covered by this standard, the material properties listed below are assumed at an operating temperature of 20°C (68°F).[1]
| Material Properties at an Operating Temperature of 20°C (68°F) | |||||
|---|---|---|---|---|---|
| Property/ Material | Tensile Modulus of Elasticity | Creep Modulus | Poisson’s Ratio | Rate of Thermal Expansion | |
| Symbol | 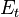
|
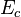
|
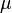
|

| |
| Units | Mpa(psi) | Mpa(psi) | dimensionless | cm/cm/°C (ft/ft/°F) | |
| ABS | 2,206 (320,000) | Varies (refer to 8.2.2) | 0.35 | 10.3 E-5 (5.7 E-5) | |
| HPDE | 862 (125,000) | 0.45 | 18.0 E-5 (10.0 E-5) | ||
| PVC | 2,827 (410,000) | 0.35 | 5.2 E-5 (3.0 E-5) | ||
| Test Method | ASTM D638 | ISO 899 | ASTM E132 | ASTM D696 | |
Material Properties of Thermoplastic Pipe
Creep modulus varies by plastic material, temperature, age, and time of loading (TI). The time of loading is defined as the period of time a plastic siphonic piping system is expected to be operating at the relevant pressure. Once the storm event is over and the system drains, the material recovers and assumes its original tensile modulus (i.e., EC(TI= 0) = Et). However, the material elastic modulus and creep modulus also typically decrease with age. Refer to pipe manufacturer data for the appropriate creep modulus value.
Calculation of allowable pressure 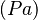 : The minimum required pipe wall thickness
: The minimum required pipe wall thickness 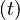 shall be based on Equations 8.1 and 8.2 in Table 8.2 and the mechanical and dimensional properties of the pipe material evaluated. Equations 8.1 and 8.2 apply for a long cyclindrical tube of length L and wall thickness t under uniform external pressure with
shall be based on Equations 8.1 and 8.2 in Table 8.2 and the mechanical and dimensional properties of the pipe material evaluated. Equations 8.1 and 8.2 apply for a long cyclindrical tube of length L and wall thickness t under uniform external pressure with 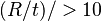 .
.
| Table 8.2 Pipe Wall Thickness Calculations | |||||
|---|---|---|---|---|---|
| Equation 8.1 When, |
Equation 8.2 When | ||||
![P_{cr}= \frac{E_c}{3(1-\mu^2)} \Bigg[ \frac{t}{R} \Bigg]^3](/images/math/a/9/e/a9eae55e231d6bffd5e002ca1ce78246.png)
|
![P_{cr}= 0.807 \Bigg[ \frac{E_c t^2}{LR} \Bigg] \Bigg[ \Big( \frac{1}{1-\mu^2} \Big) \Big( \frac{t}{R} \Big)^2 \Bigg] ^{0.25}](/images/math/8/5/5/8559cae769ac49b70a6cc937970a6a3e.png)
| ||||
When, 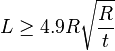
|
When, 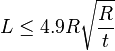
| ||||
The parameter 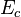 is the elastic creep modulus at the specified time of loading. The allowable pressure
is the elastic creep modulus at the specified time of loading. The allowable pressure 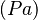 shall be the calculated critical buckling pressure divided by the factor of safety (FS) as in equation below:
shall be the calculated critical buckling pressure divided by the factor of safety (FS) as in equation below:
Equation 8.3:
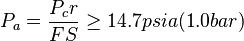
- Poisson's ratio is the ratio of the relative contraction strain (or transverse strain) normal to the applied load - to the relative extension strain (or axial strain) in the direction of the applied load [2]
Poisson's Ratio can be expressed as;
μ = - εt / εl where μ = Poisson's ratio εt = transverse strain εl = longitudinal or axial strain
Strain can be expressed as;
ε = dl / L where dl = change in length (m, ft) L = initial length (m, ft) For most common materials the Poisson's ratio is in the range 0 - 0.5. μ = Poisson’s Ratio (dimensionless)
- Poisson's ratio describes the relative change of the lateral dimension of an object when the load is applied on the longitudinal direction and vice versa. (See reference video link [1])
References
- ↑ ASPE Standard 45
- ↑ http://www.engineeringtoolbox.com/poissons-ratio-d_1224.html